There is hardly any domain where game theory doesn’t find an application. We are living through a time when collaboration and competition go hand-in-hand. A time when rational individuals make strategic decisions for collective goodness. Though based on mathematical models, game theory has application in strategic decision making. It’s about making decisions while keeping in mind the actions that the competitors will take. Game theory in blockchain technology has a dual role to play i.e. inward & outward. Inward is to apply the principles of the theory to the blockchain protocol. Outward is to integrate the strategic decision-making model with the business’ process.
The Prisoner’s Dilemma: Trust & Transparency
The central dilemma in the blockchain is that you don’t know who knows
Trust & Transparency are the two pillars that define blockchain. It utilizes transparency in the process to establish trust among the ecosystem participants. The logic of trust & transparency comes from one of the most known experiments of the game theory i.e. “The Prisoner’s Dilemma“. Let’s understand the experiment first.
Two prisoners were brought in for questioning for their involvement in the same or similar crime. The punishment for this crime is three years. However, police suspect both prisoners of collaborating to commit a more serious crime. The punishment for this crime is five years. Police had no witness to prove their involvement in the crime. Hence, the police decided to offer a deal. The deal is if one prisoner confesses to the more serious crime and indicates the involvement of the other then one who confesses will get a punishment of two years and then another prisoner will get a severe punishment of nine years. If both prisoners confess then each gets a standard punishment of five years. Let’s name the prisoners as A & B
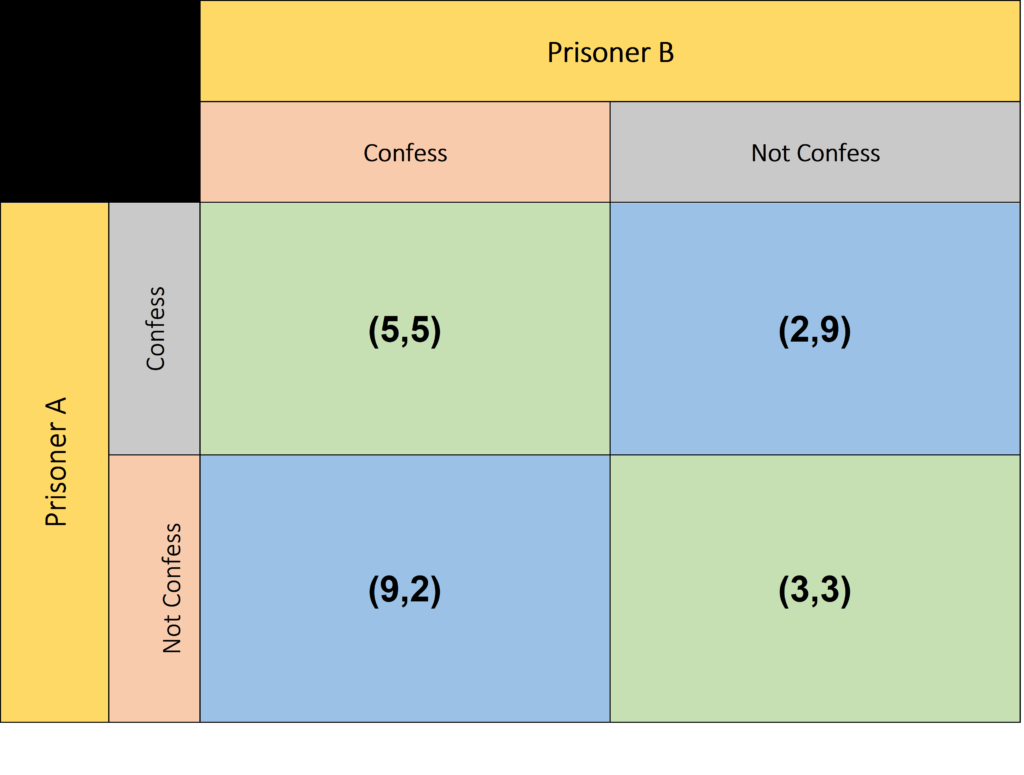
The best option for both prisoners is to stay silent and not confess. However, they choose to confess as neither of the two is sure of the other’s strategy. This is what is called the Nash Equilibrium.
Blockchain is secure because the entire protocol is based on the Nash Equilibrium
Operational process across industries are mired with similar situations. Trust among the operations value chain participants is non-existent and transparency has become translucent. This has resulted in reaching a Nash Equilibrium among various spheres of the business, causing inefficiency and loss of capital due to various types of cost. This is where blockchain comes into the picture. It can infuse transparency & trust among the ecosystem participants and can eliminate the middle-men and cost associated with them.
What Are The Various Models of The Game Theory
Non-Cooperative Game
In a non-cooperative game, there shouldn’t be any communication of the strategies to form a co-operation. The strategy chosen by any player in a non-cooperative game should be impetuous and rational. Let’s try to understand how does that work in a blockchain.
In a blockchain network, miners compete for the reward by investing strategically into the computational power. Non-Cooperative games could be used as an underlying model for miner’s interaction. In any given situation miners will work their strategies to maximize the reward i.e. utility. A Nash Equilibrium is achieved where no miner can gain more reward by modifying its strategy when the strategy of other miners is unchanged. The non-cooperative theory could be applied to scenarios like managing users interaction, fork selection, etc.
Extensive Form Game
Unlike non-cooperative games, extensive form games are dynamic in nature wherein strategies are made as per a pre-defined order. The non-cooperative theory could be applied to both static and dynamic games. In extensive form games, interactions are represented as a game tree with rewards mentioned at the end of the branch. A non-cooperative game comprises of various sub-games. The Nash Equilibrium, in this case, is achieved by applying the principles of reverse engineering or induction.
In a blockchain, extensive form model could be applied to optimize the mining rewards, cheating among the users, etc.
It’s about anticipating the decisions of the last move and working backward to the first move
Stackelberg Model
The Stackelberg model works on the principle of “Leaders” and “Followers“. The followers decide their strategies based on the decisions taken by the leaders. All the participants are rational and work towards maximizing their utilities. The model could be applied in a scenario involving two or more than two ecosystem participants. Let’s assume a blockchain system comprising of a service provider and various miners. The service provider offers computational power to the miners in exchange of price. The miners optimize their demand to maximize rewards. The service provider acts as a leader by setting the price and the miners, as a follower, design their strategy accordingly.
The Nash Equilibrium in the Stackelberg model is reached through backward induction method
Stochastic Game
A collection of several repetitive, static non-cooperative games is known as stochastic game. In the stochastic game, players change their strategies based on past behaviors & approach. The stochastic model could be applied to analyze the chain selection, security issues, etc. The end outcome of the stochastic games is known as “Markov Perfect Equilibrium” (MPE).
The Nakamoto Protocol i.e. mining on the longest chain is an example of the Markov Perfect Equilibrium
What’s Next?
- Application of game theory in blockchain security
- Application of game theory in mining
- Application of game theory in various use cases
[cp_modal id=”cp_id_3e64e”] [/cp_modal]




Informative